An arc function is an inverse function, a function which undoes the work of another function. Examine inverses with inverse.gsp.

      Strictly speaking, the symbol sin^-1( ) or Arcsin( ) is used for the Arcsine function, the function that undoes the sine. This function returns only one answer for each input and it corresponds to the blue arcsine graph at the left.

      Arcsine may be thought of as "the angle whose sine is" making arcsine(1/2) mean "the angle whose sine is 1/2" or π/6.

      The symbol sin^-1( ) is used often when one wishes more than one or even all the values possible even though these values are not covered by the Arcsine function. See below for a better understanding of this.

      Think of the Arcsine as the principal arcsine.

      A function can only be an inverse if it is 1-to-1 and undoes exactly the desired function. See inverse function notes for a review of inverse functions.

      In the graph at the left, notice that the sine function, pink and dashed, is not 1-to-1 because it is periodic and repeats every 2π.

      The only way for f(x) = sin(x) to undo g(x)= sin^-1(x) is if it is 1-to-1 which requires the domain to be restricted.

      Once this is done f(x) = sin(x) undoes g(x)= sin^-1(x) and g(x)= sin^-1(x) undoes f(x) = sin(x).

      If we only use the pink part of the sine curve, from x-values of - π/2 to + π/2, then the restricted sine is reflected over the line y=x to take the inverse graphically, the inverse, g(x) = sin^-1(x) is found and will indeed give us a single value for every x value from -1 to 1, inclusive.

      This restriction makes the domain of the Arcsine -1< x < 1 and the range - π/2< y <+π/2; as needed.

      A "negative first quadrant angle" is not proper mathematical terminology, but, it is a very useful way of speaking about the first quadrant of standard position angles spun in a clockwise direction, making their measures negatives.

      The arcsine of a positive number is a first quadrant angle, sin^-1(+) is in quadrant I.
      The arcsine of zero is zero, sin^-1(0) is 0.
      The arcsine of a negative number is a negative first quadrant angle, sin^-1(-) is in quadrant -I, a clockwise-angle of less than or equal to - π/2.

      The arccosine of a positive number is a first quadrant angle, cos^-1(+) is in quadrant I.
      The arccosine of zero is π/2, cos^-1(0) is π/2.
      The arccosine of a negative number is a second quadrant angle, cos^-1(-) is in quadrant II.

      The arctangent of a positive number is a first quadrant angle, tan^-1(+) is in quadrant I.
      The arctangent of zero is zero, tan^-1(0) is 0.
      The arctangent of a negative number is a negative first quadrant angle, sin^-1(-) is in quadrant -I, a clockwise-angle of less than - π/2.

      Though the Arcsine function is used to solve the equation, solutions to the equation may not be in the range of the arcsine function.

      The gray box below lists the kinds of triangles one may "solve" -- figure out the measures of all the angles and side.

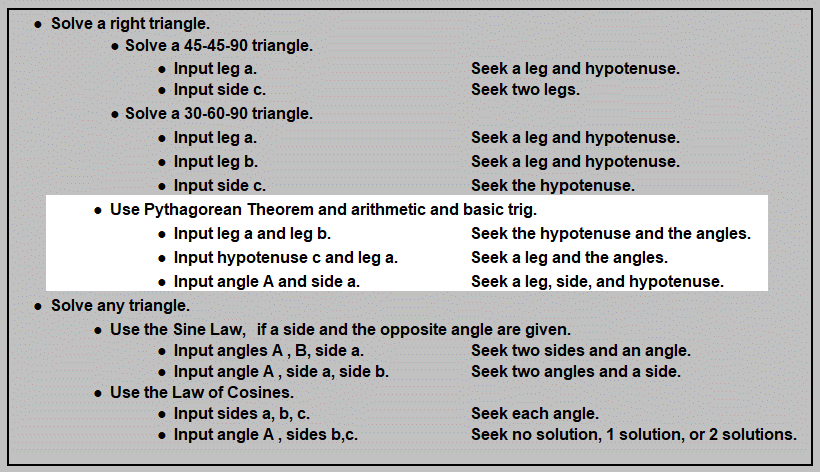
      Because you, the reader, have already studied the 45-45-90 and 30-60-90 right triangles and know the lengths of their sides and how similar figures work, you have enough information to solve triangles listed above the white box.

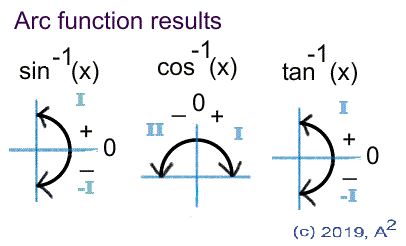
      Through work on this page and arcfunctions, the number of right triangles that may be solved is greatly increased.  Below the white box are listed the triangles for which more math is required to solve the triangle, the triangles which may not be right triangles .But, now we address solving right triangles using arcfunctions.

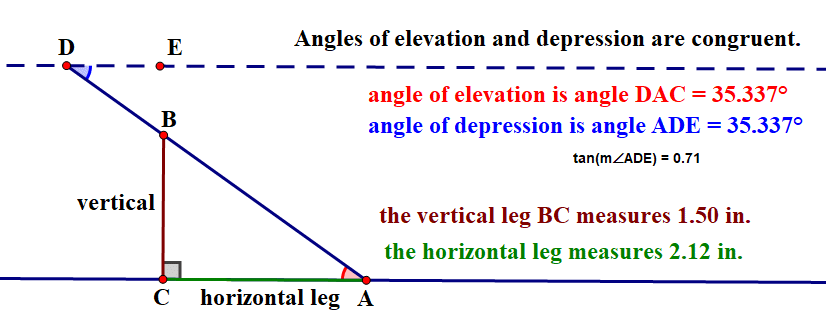
The situtation usually studied include:
• a ladder leaning against a building,
• a pole casting a shadow,
• a tree of unknown hight but with a known angle of elevation a distance from the tree
• a right triangle in which the lengths of the sides are known but the angles are not known,
• a pond the width of which is to be measured
• an air plane at a known altitude which is to hit a target a distance away from the plane
• a triangle in which some measures are known and some are not, but, all measures are needed

Q1.       The world's steepest road is CA-108, along the Sonora Pass, in California. Its grade, its rise/run of 13:50, stated as a percent is 26%. What incline, angle of elevation is this? (Source: https://matadornetwork.com/read/steepest-highway-grades-in-the-us/)

Q2.       Solve the triangle with C = 90°, a = 2.5, c= 6.

Q3.       Complete from Precalc Notes, page "To Be Printed" 27. pdf of use arcfx to solve triangles con-dots.

Q4.       Write and solve a word problem similar to Q1 using a "situtation usually studied" as listed above.