|
Sometimes it's easy to graph by inspection. Sometimes it's not.
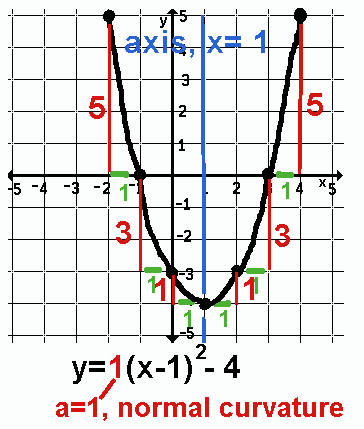
If the quadratic is written as y = a(x - h)² + k, it's easy.
If the quadratic is written as y = ax² + bx + c, it's not as easy.
Two "shifts" are stated in y = a(x - h)² + k.
There is a "
domain shifts" forced by the (x - h). It
positions the curve left and right. There is a "
range shifts" forced by the + k. It positions
the curve up and down. 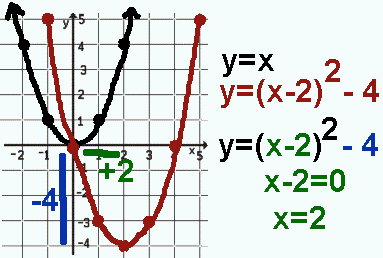
See
Composition of Functions and the how functions are created.
See the graph of
y = (x - 2)² - 4 from a
composition of functions standpoint.
See
Curve Shifting and this graph and function explained in detail.
In the curve y = (x - 2)² - 4, the squaring, symbolized by 1()² gives the
curve its shape and dilation. The (x-2) "recenters" the curve horizontally from a axis of symmetry of x=0 to
an axis of symmetry of x-2=0 or x=2. The - 4 "shifts" the curve down 4.

The graph y=(x-3)²-1 is shifted to the right 3 and down 1 compared to the curve
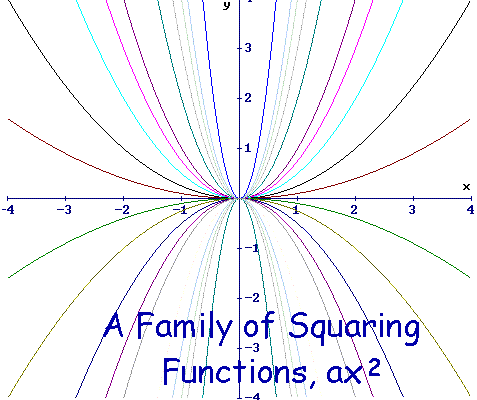
y=x². The graph y=2(x-2)²+1 is dilated and made
steeper or "skinny" and shifted right 2 and up 1. The graph of
y=-(x-3)²+4 is concave down with normal dilation, shifted to the right 3, and shifted up 4.
| 








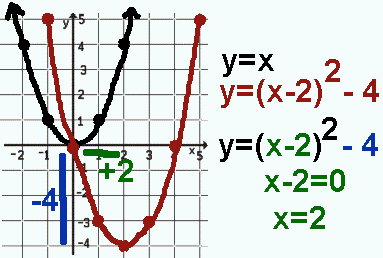


![[MC,i. Home]](http://www.mathnstuff.com/math/spoken/here/1gif/mcihome.gif)
![[Words]](http://www.mathnstuff.com/math/spoken/here/1gif/words.gif)

![[this semester's schedule w/links]](http://www.mathnstuff.com/gif/semestr.gif)
![[Good Stuff -- free & valuable resources]](http://www.mathnstuff.com/gif/goods.gif)