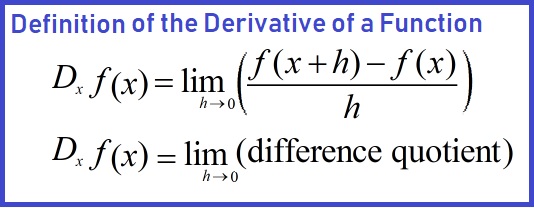


|
| Vocabulary and Basics First
Before one can take a derivative by taking the limit of a difference quotient, one needs to know what a difference quotient and a limit are. Difference is a subtract expression, as in the difference between two numbers, as in the difference between 9 and 7 is 9 - 7 or 2. The difference may be between a function at one value of x and the function of another value of x is f(x2) - f(x1). To find a difference, just subtract.
Quotient is a division expression, as in the result when two numbers are divided, as in the quotient 8 ÷ 2 is 4. To find a quotient, divide.
Remember also that a division expression may be written as a fraction, as in,
numerator /
denominator,
A difference quotient is a fraction (a division expression) in which the numerator is a difference in function values and the denominator is a difference in x values -- JUST LIKE SLOPE! To take a limit, think "approach." Use the sketchpad in the box to examine "approach."
Click to enlarge or isolate an image. |

| Some Examples | ||
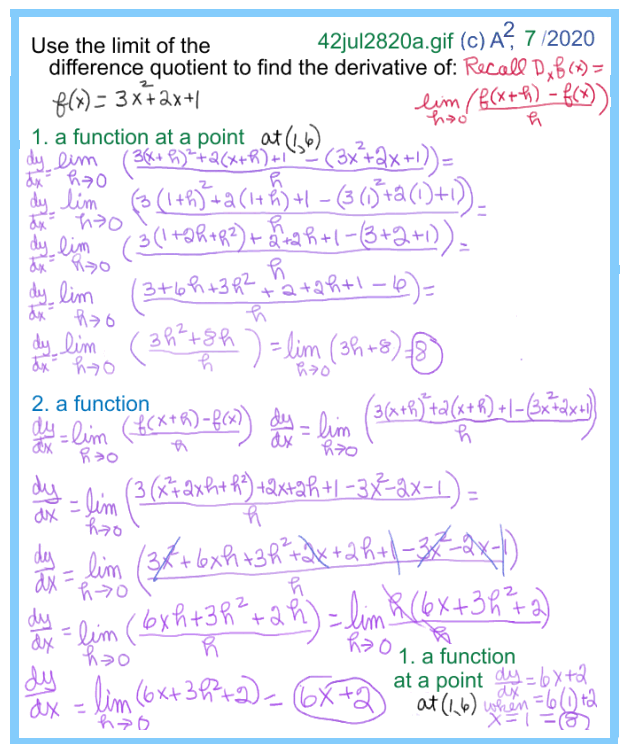
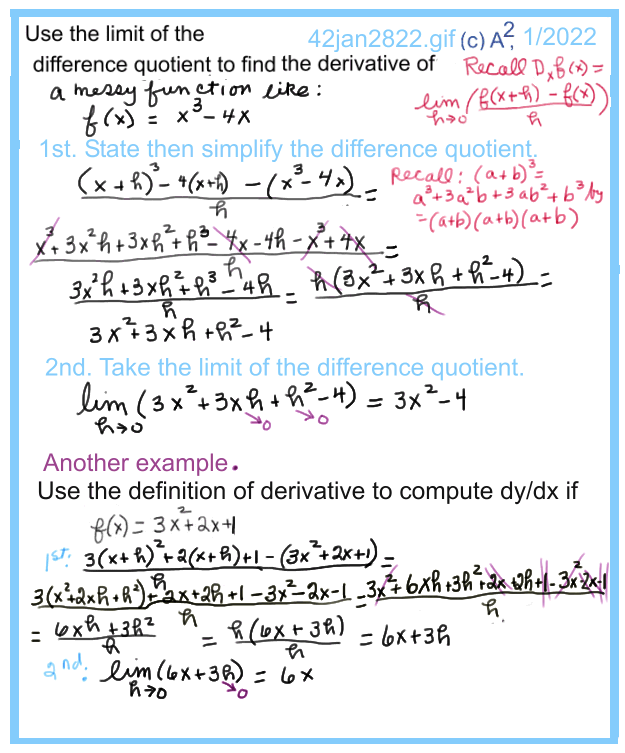 |
Derivative Proofs
| |

www.mathnstuff.com/math/spoken/here/2class/420/byLimOfDifQ.htm |