Among Basic Functions

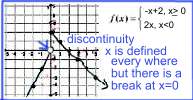
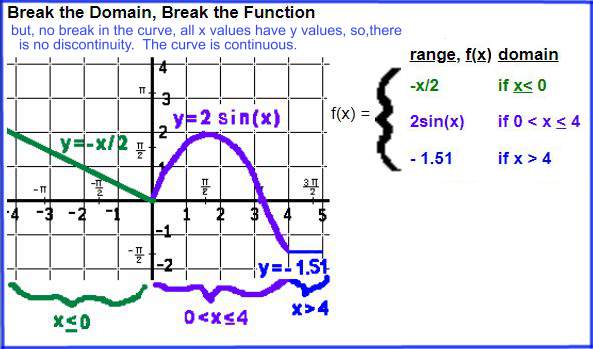
Among the basic functions, the greatest integer functions and reciprocal functions are the only ones with a discontinuity. The discontinuity in the reciprocal function f(x) = 1/(x+2) is because one may not divide by 0 and that would happen if x equaled -2. The line x = -2 is a vertical asymptote so the curve is "broken" there. With the greatest integer function, there are multiple discontinuities. A discontinuity exist everytime x is an integer. For x values greater than or equal to the integer, the graph goes on the "higher step." For x values less than the integer, the graph goes on the "lower step." |



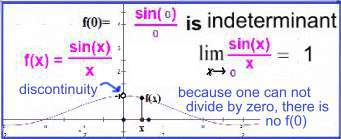
 0(sin(x)/x).
0(sin(x)/x).![[MC,i. Home]](http://www.mathnstuff.com/math/spoken/here/1gif/mcihome.gif)
![[Table]](http://www.mathnstuff.com/math/spoken/here/1gif/table.gif)
![[Words]](http://www.mathnstuff.com/math/spoken/here/1gif/words.gif)

![[this semester's schedule w/links]](http://www.mathnstuff.com/gif/semestr.gif)
![[Good Stuff -- free & valuable resources]](http://www.mathnstuff.com/gif/goods.gif)
![[next]](http://www.mathnstuff.com/math/spoken/here/1gif/next.gif)
![[last]](http://www.mathnstuff.com/math/spoken/here/1gif/last.gif)