The product of the sum and the difference of two numbers is the difference of their squares.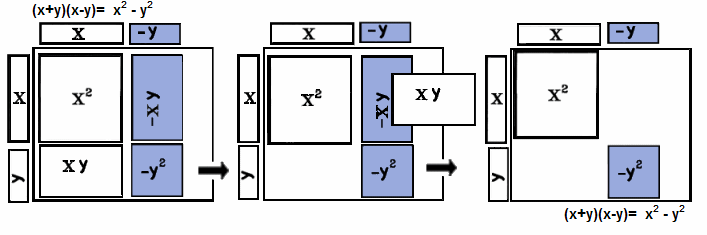

      What product, if any, produces the difference of two cubes?

      This is a division (easier) or a factoring (harder) question and involves third-degree terms or prisms.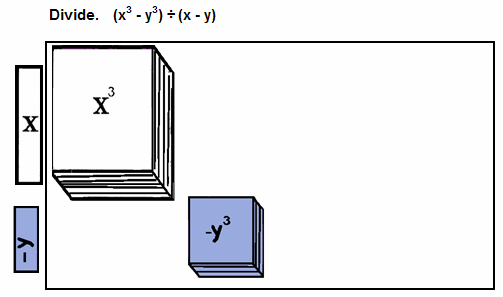

      The quotient contains a y² term and an x² term. The dividend require zeros to fill the required areas.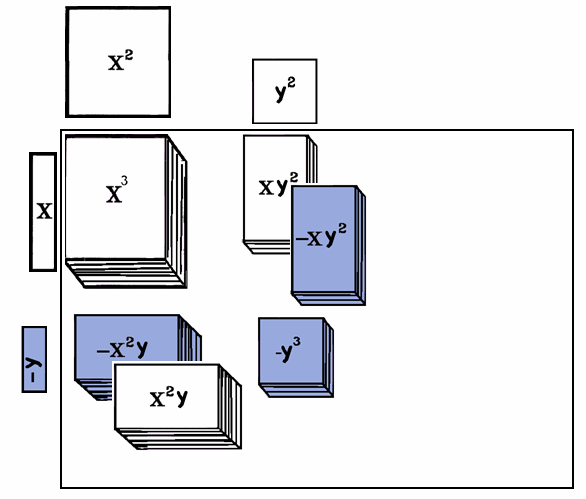

      Two different zeros are required. One is xy² - xy² and the other is x²y - x²y.

      Is it possible to rearrange all the required terms to the factor the difference of two cubes?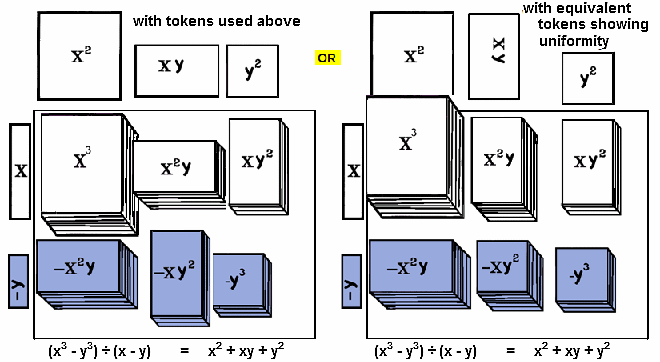

      The difference of two cubes equals the product of the difference of the numbers and the sum of their product and their squares.

      The next logical question is "What does it take to factor the sum of two cubes?" if it is possible to achieve that task.

      Review what is known. Look for a pattern. Factor and fill in the box.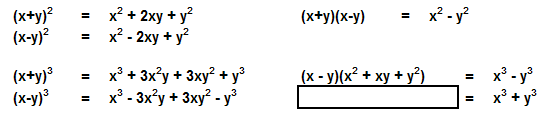

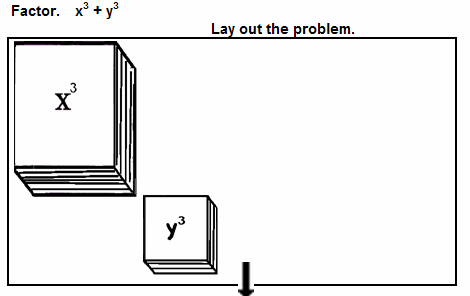
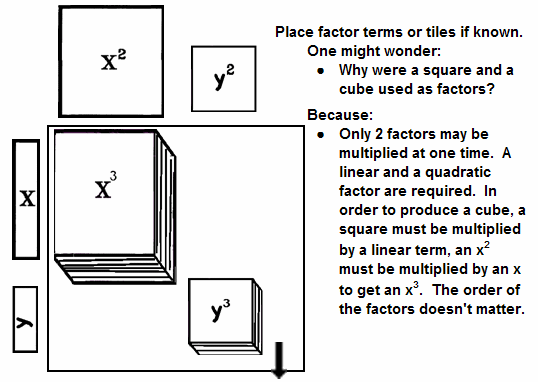
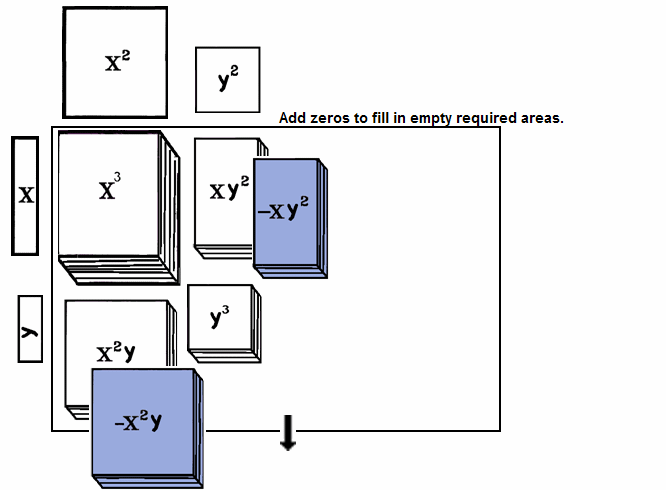
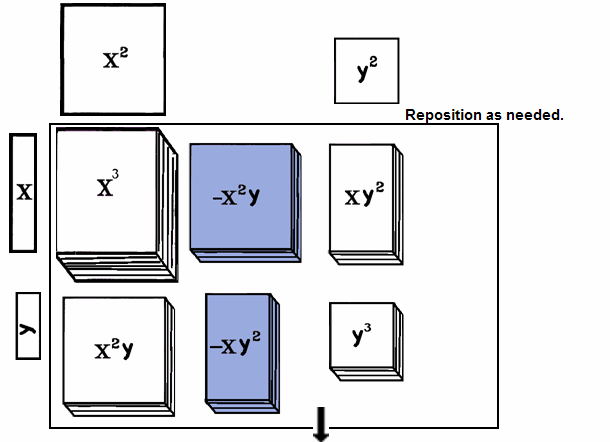
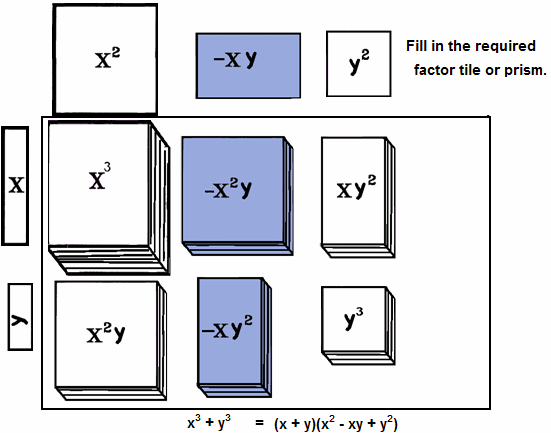
      The sum of two cubes equals the product of their sum and the sum of their squares decreased by their product.

      These Special Products summarize what is known. In other factoring, look for a pattern and factor.

      The sum of two squares is not factorable over the reals. Complex number are required. See Got Your Number -- Number Systems & Computation for more info.