|
Binomial Squared and Cubed |
|
The
squares of
binomials are sometimes addressed manipulatively. The
cubes of binomials are almost never addressed manipulatively
or in this depth. Term Tiles and, in particular, Tokens permit this presentation.
There is insight to be gained through these tokens, even for the teacher.
A binomial squared is a prefect
trinomial square. Any binomial may be squared,
but the square of the sum of two numbers and the square of the difference of two numbers are
important for the patterns they display.
A binomial cubed takes a binomial squared and multiplies it by the binomial.

Above, the tiles and tokens have been oriented and labeled to facilitate verification
of the multiplication. Notice that there are two groups of three token
which have equivalent volumes and shapes, but, which appear very different.
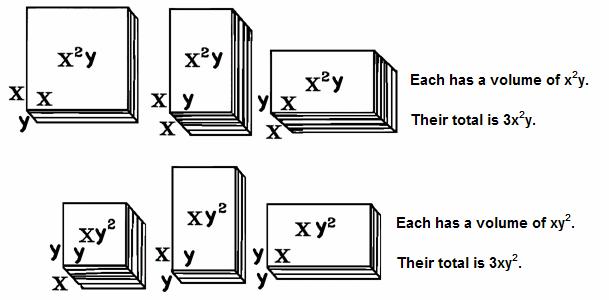
One more orientation is important to examine. The cube of the sum is really a
cube with each side the length of the sum. The following examines the 2 layers of
prisms required by the multiplication which creates the cube
of the sum.

The cube of the difference of two numbers is very similar. Take the square of the difference and multiply it by the difference.
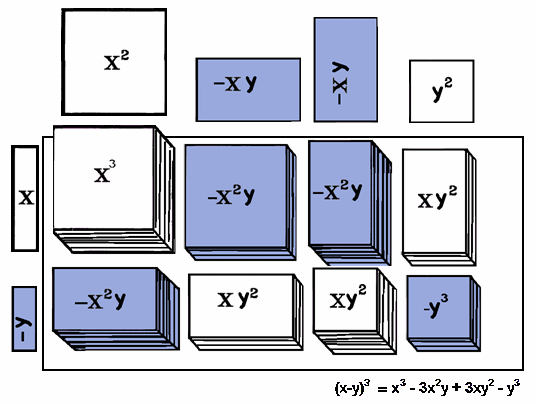
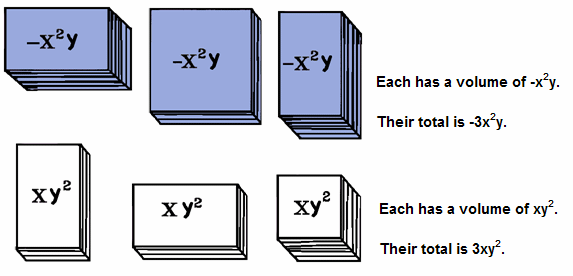
Shown below, the cube of the difference of two numbers is displayed in layers
and as a cube of "negative and positive volumes."

| The Squares and Cubes of the Sums and
Differences of Two Numbers | |
| 









