Tilted Tile Format is used only if all terms are of the same dimension or power --
all constant terms or all linear terms or all quadratic terms, for example. Tilted Tile Format may not be used if
any binomial is a part of the fraction.
It is best used to explain multiplication, and later division, of fractions and this is
done in 4th, 5th, and 6th grades with constants.
Multiplication of Proper Fractions in Tilted Tile Format
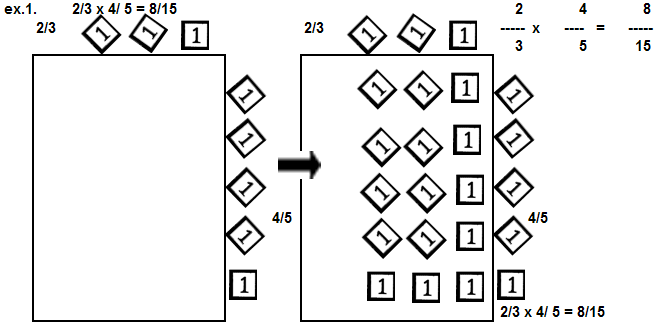
| 1st: | Represent the first fraction in storage horizontally on the top in the following way.
Let the denominator give you the number of tiles to be used in each display area.
The denominator names the size.
Let the numerator give you the number of tiles to tilt at an angle to the edge.
The numerator names the number of fractional pieces. |
| 2nd: | Represent the second fraction in storage vertically on the right in the following way.
Let the denominator give you the number of tiles to be used in each display area.
The denominator names the size.
Let the numberator give you the number of tiles to tilt at an angle to the edge.
The numerator names the number of fractional pieces. |
| 3rd: | Multiply. Fill the display area matching top tile to side tile in the following way.
TILT ONLY the tiles which are TILTED on the top AND ALSO TITLED on the side. |
| 4th: | Count all of the tilted tiles in all of the display area(s). This is the numerator of the product. |
| 5th: | Count the number of tiles in any one of the display area(s). This is the denominator of the product. |
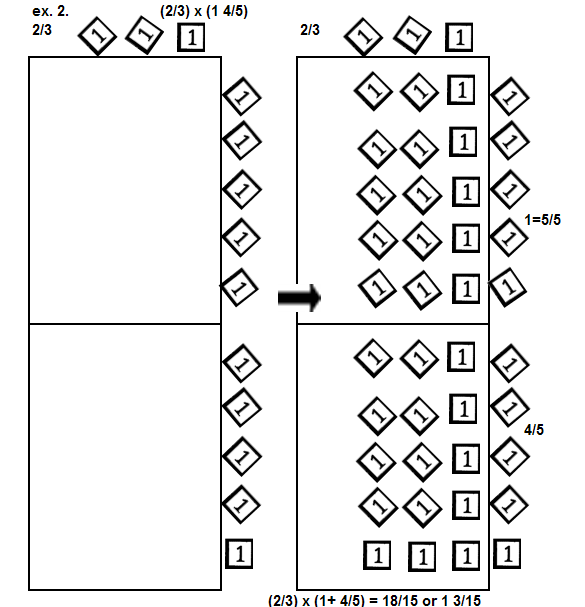
 Multiplication by an Improper Fraction Using Tilted Tile Display.
Multiplication by an Improper Fraction Using Tilted Tile Display.
| Start | More than one display area is required.
Each whole number and fraction requires on an edge, requires its own area.
The final display areas must form a rectangle. |
| 1st: | Represent the first factor in storage horizontally on the
top in the following way.
Let the denominator give you the number of tiles to be used in each
display area.
The denominator names the size.
Let the numerator give you the number of tiles to tilt at an angle to the edge.
The numerator names the number of fractional pieces. |
| 2nd: | Represent the second factor in storage vertically on the
right in the following way.
Let the denominator give you the number of tiles to be used in each display area.
The denominator names the size.
Let the numerator give you the number of tiles to tilt at an angle to the edge.
The numerator names the number of fractional pieces. |
| 3rd: | Fill the display area matching top tile to side tile in the
following way.
TILT ONLY the tiles which are TILTED on the top AND
ALSO TITLED on the side. |
| 4th: | Count all of the tilted tiles in all of the display area(s).
This is the numerator of the product. |
| 5th: | Count the number of tiles in any one of the display areas.
This is the denominator of the product. |

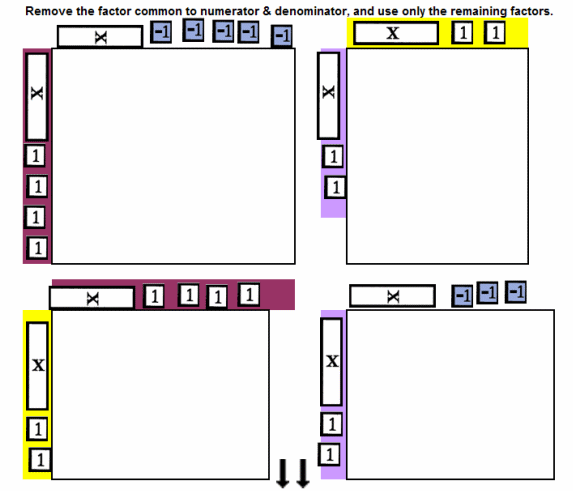
| In Numerator / Denominator Format
| |
Multiply Two Fractions in Numerator / Denominator Format
| 1st: | Use two display areas for each fraction.
Let the ones on the tops (further from the user) be for the numerators.
Let the ones on the
bottoms (closer to the user) be for the denominators. |
| 2nd: | Represent the numerators in tops or uppermost display
areas. | | 3rd: | Represent the denominators
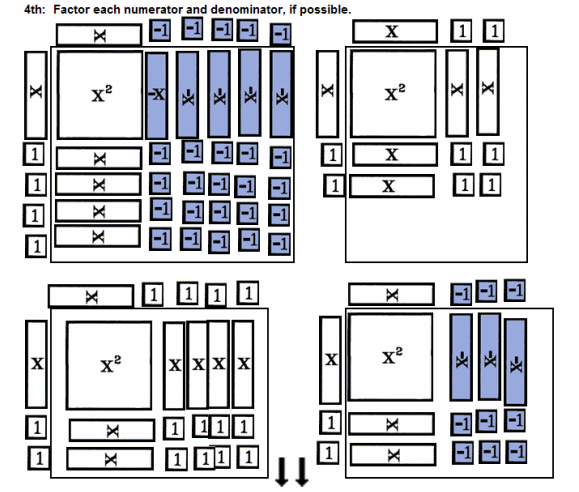
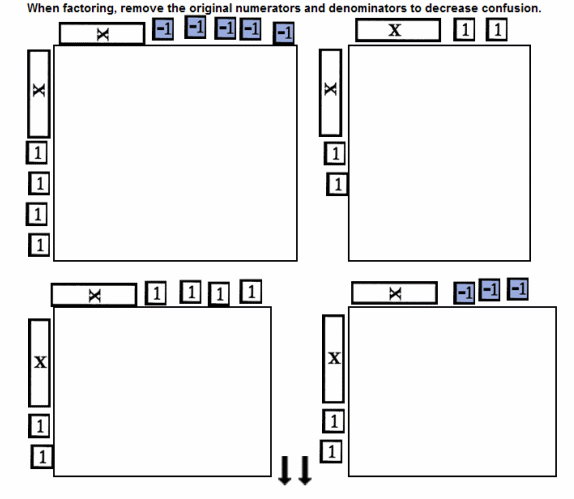
in bottoms or lower display areas. | | 4th: | Factor
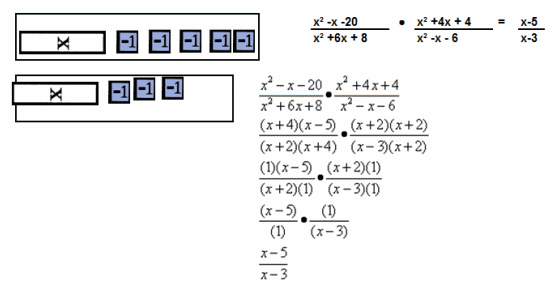
each numerator and denominator, if possible. |
| 5th: | Use the factors they DON'T have
in common as the new numerator and the new denominator.
Whenever possible, remove a top factor with an identical bottom factor.
TO REDUCE, USE THE
FACTORS
THEY DON'T HAVE IN COMMON
AS THE NEW NUMERATOR AND THE NEW DENOMINATOR.
|
|
| 6th: | The product of the numerators is the numerator of
the product, the answer. |
| 7th: | The product of the denominators is the denominator of the
product, the answer. |

|




