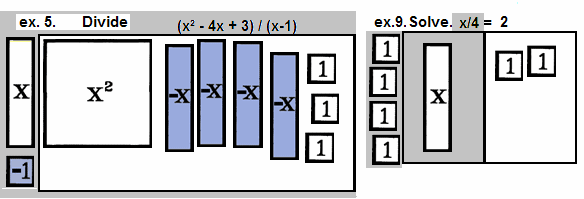
|
Again the reader is reminded "Manipulatives are made to be outgrown."
Proportions provide the perfect example. Don't use manipulatives to solve problems which may be
completed mentally. (Two kinds of mental computation are presented below.)
It is important to use previously used formats so the new material follows from the
old. In division and equation solving, the divisor or denominator goes in the VERTICAL STORAGE AREA. The
stage has been set. Use the display area and tiles to signify fractions and to solve proportions.
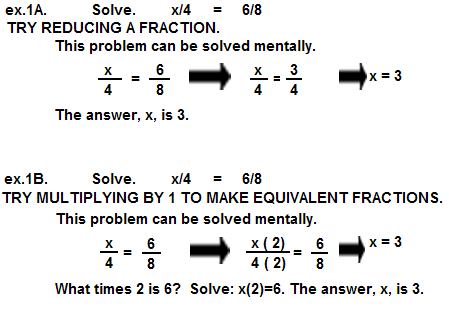
To solve easy proportions, complete the problem mentally. For more
sophisticated problems, CROSS MULTIPLY and remember to check for extraneous roots.
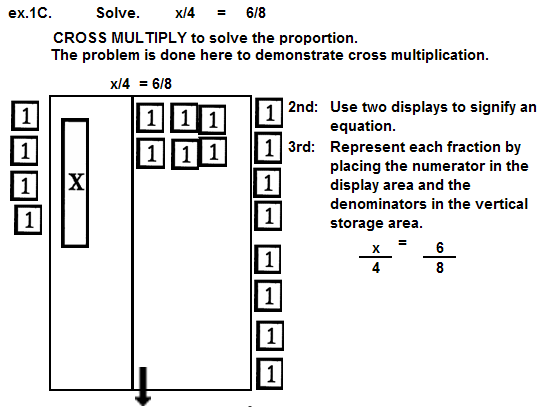
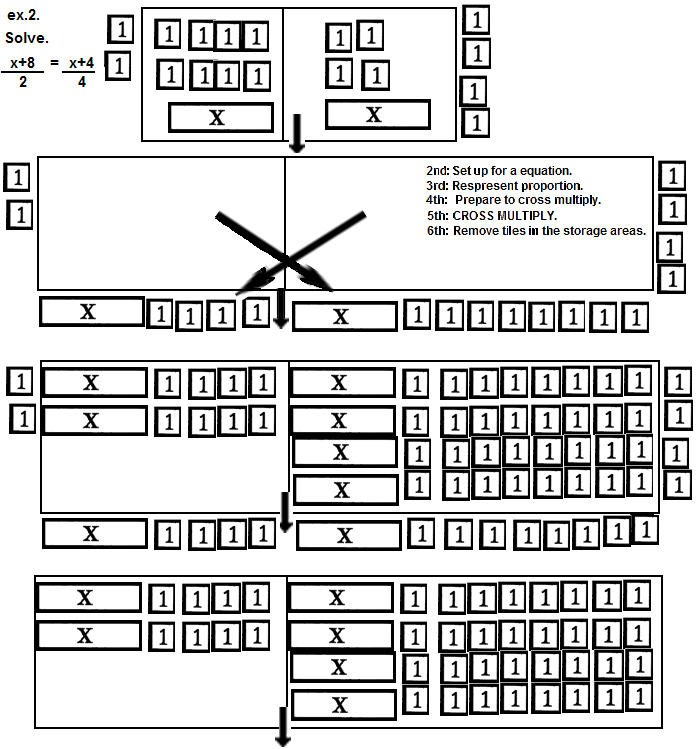
Solve A Proportion.
| 1st: | Attempt to solve the proportion using mental
computation including reducing a fraction or multiplying by 1. |
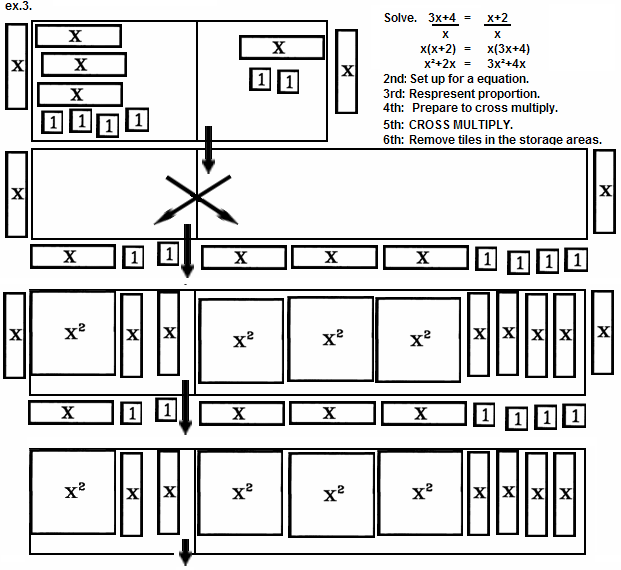
| 2nd: | Use two displays to signify an equation. |
| 3rd: | Represent each fraction placing the numerator in the
display are and the denominators in the vertical storage area.
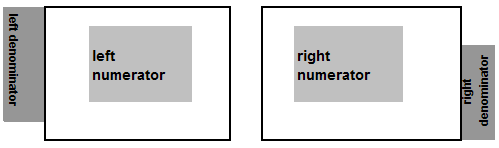 |
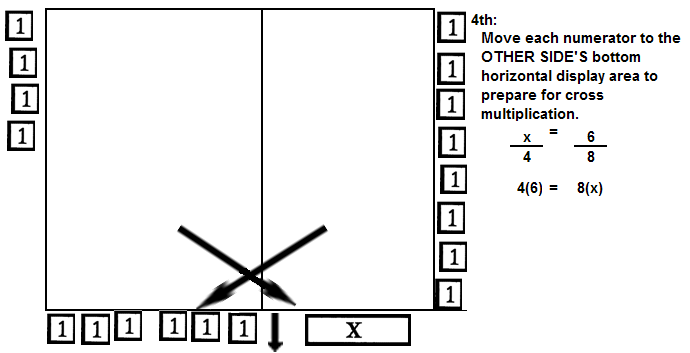
| 4th: | Move each numerator to the OTHER SIDE'S bottom
horizontal display area to prepare for cross multiplication.
 |
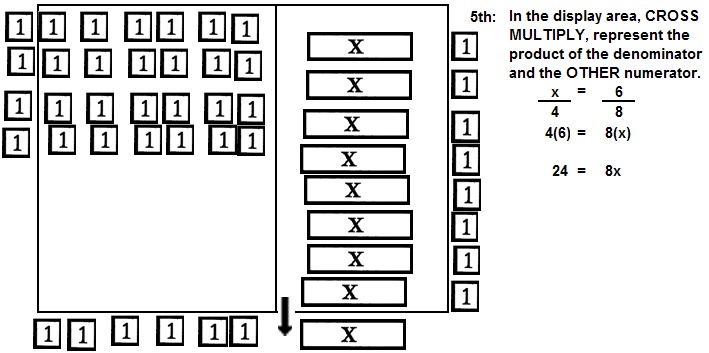
| 5th: | In the display area, CROSS MULTIPLY, represent the
product of the denominator and the OTHER numerator. |
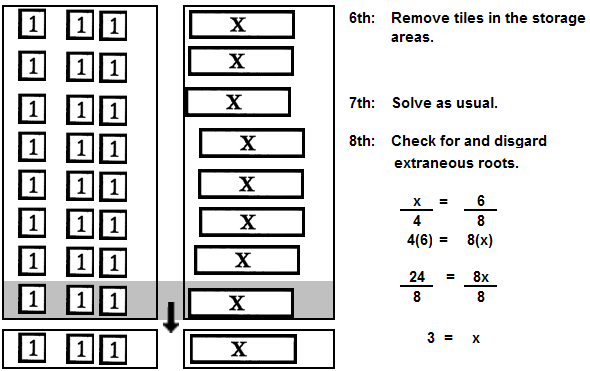
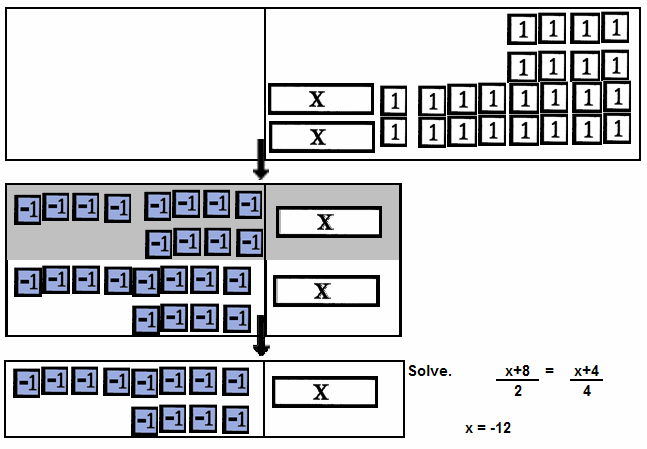
| 6th: | Remove tiles in the storage areas. |
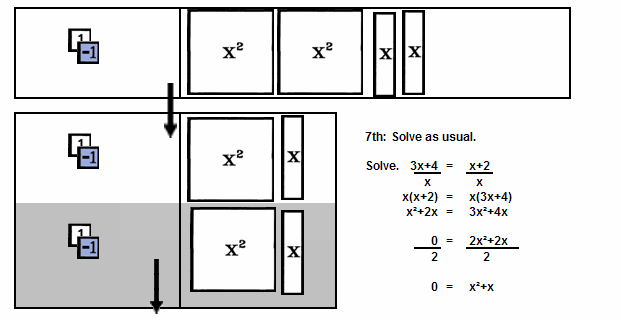
| 7th: | Solve as usual. |
| 8th: | Check for and discard extraneous roots. |
|
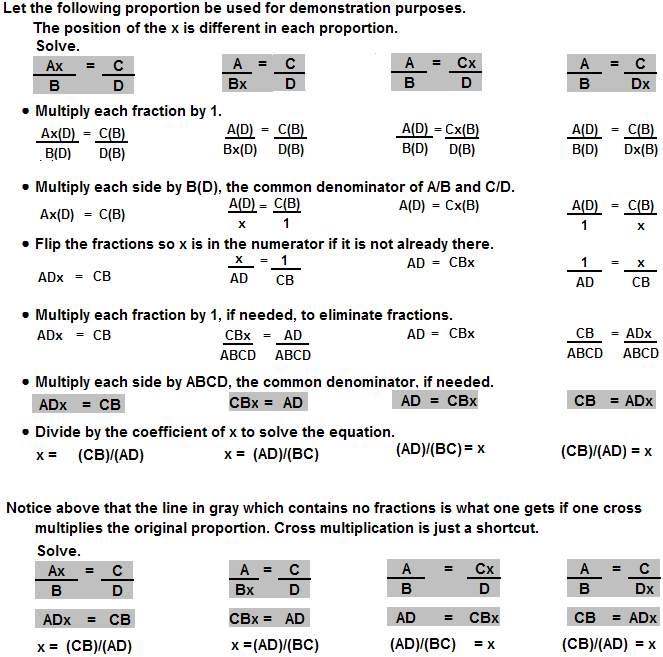
Remind me. Why Is It Legal to Cross Multiply? | |
- It is legal to multiply any number by 1 without changing its value.
- In solving an equation you must do the same thing to each side of the equation.
- If you "flip" the proportion, exchanging numerators with denominators, the proportion is still true.
This is the same as taking the reciprocal of each side of the equation.
|
|
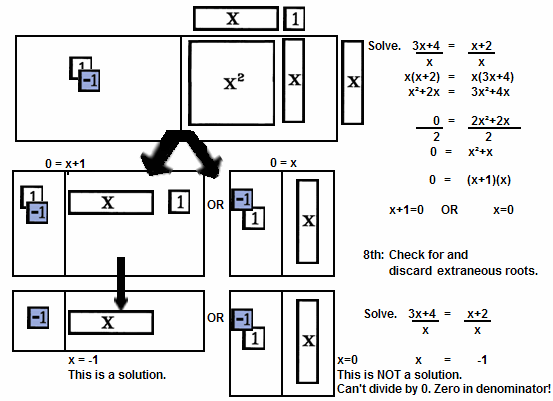
| Check for extraneous roots.
| |
Did you notice the extraneous root in the 8th, last, step of the example 3, just
above. The problem resulted in 2 solutions and THIS IS PERFECTLY CORRECT. One of the solutions
would have meant a 0 in the denominator of a fraction and THIS IS NOT MATHEMATICALLY CORRECT. The
result is an extraneous or extra root.
|




