|
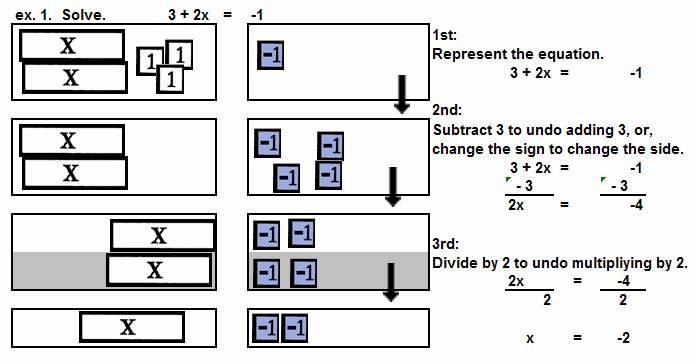
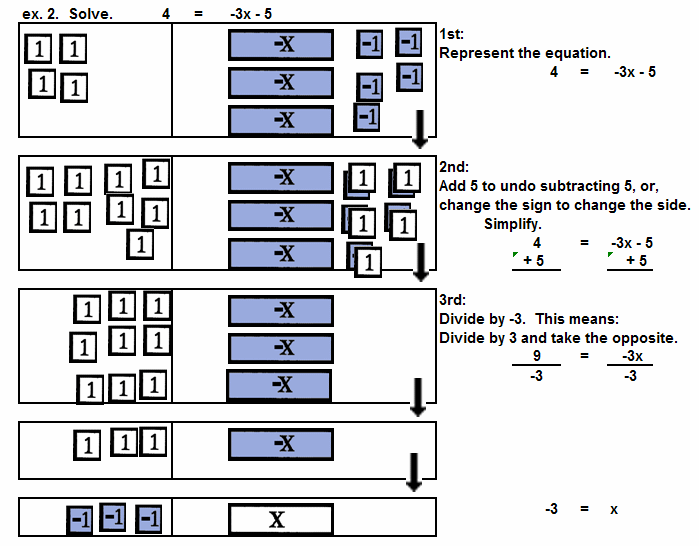
To solve a linear equation with more than one operation, one must undo the
operations in reverse of the order of operations.

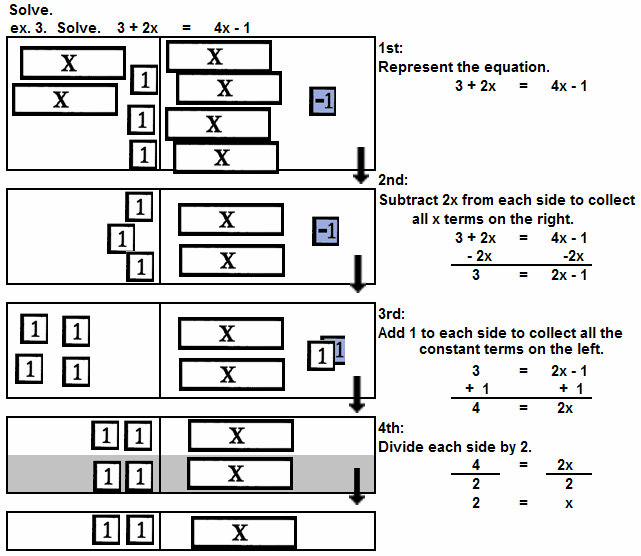
| Solve an equation with a binomial on each side.
| |
This is the HARDEST KIND OF LINEAR EQUATION TO SOLVE. It is the hardest
because the solver has the most freedom and an insecure student is less confident with more freedom.
Addition and subtraction must be undone before multiplication or division is
undone, and the goal is still to put all the x terms on one side. But, there are 4 ways in which this may
productively be done.

Which way should one choose? Though it doesn't matter, choose first to MOVE
ALL THE X TERMS TO THE SIDE WITH THE LARGEST X TERM at the start of the problem. Once the x terms
are combined, add or subtract to combine the constant terms.
Solve a Linear Equation.
| 1st: |
Distribute and combine like terms on each side of the equation to simplify
each expression. |
| 2nd: |
Add or subtract to collect all the x, linear, terms to one side of the equation. |
| 3rd: |
Add or subtract to collect all the constant terms on the other side of the equation. |
| 4th: |
Multiply or divide to make 1 the coefficient of the x, linear, term. |
| 5th: |
(Optional) Check the solution. |
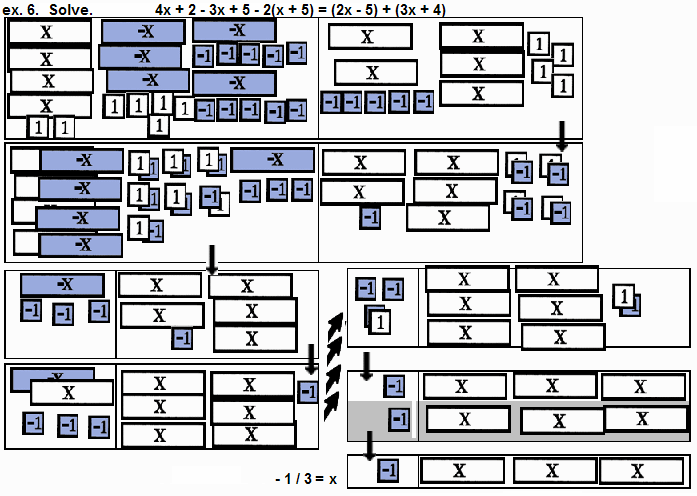
| Solve an equation with complicated
expressions on each side. | |
Which way should one choose? It doesn't matter. These problems are not as
hard as the ones just completed. As suggested above, move all the x terms to the side with the largest x term
at the start of the problem. Once the x terms are combined, add or subtract to combine the constant terms.
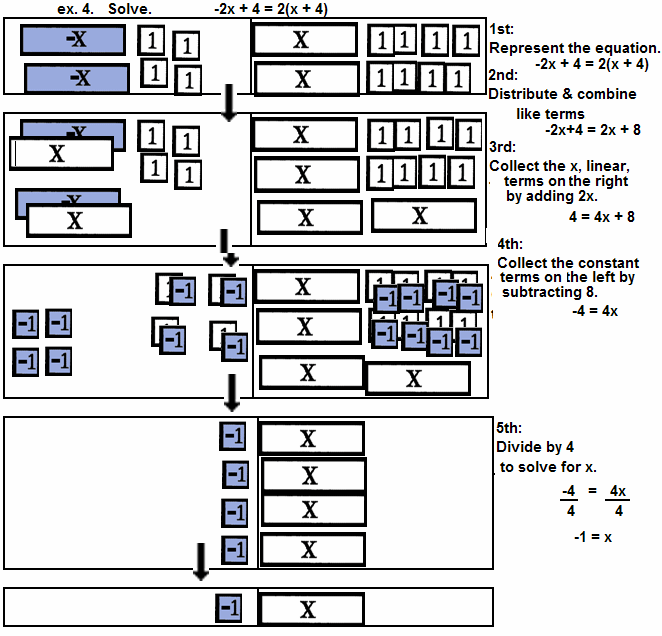

The following example illustrates how much work might be necessary in order
to simplify each side BEFORE "moving" terms from one side to another begins.
| 



