
The Visual / Auditory / Symbolic / Kinesthetic Approach to Algebra

In math, as in English, AND is a signal that AT LEAST TWO IDEAS are involved. These ideas may involve addition, but not always. In math, as in English, OF is a signal that information about ONE ITEM is going to be stated. In the above examples, the ONE ITEM is the "product" or the "sum" or the "area." The information about each respective item is the "five and seven" and the "five and seven" and the "two and a number" and the "length and width." The OF signaled that the information was going to be stated. 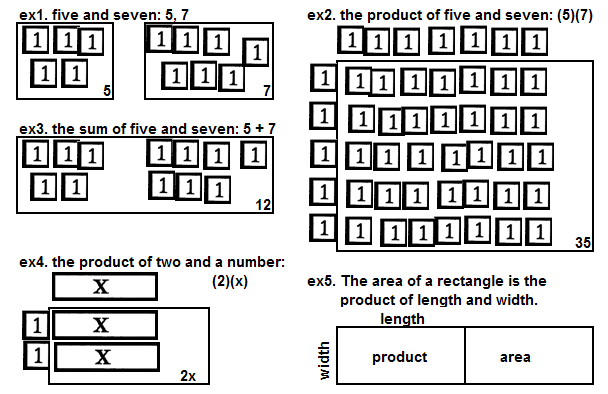
Many of those situation can not be represented manipulatively by these tile and tokens, but, may be represented by more sophisticated manipulatives not covered by this text. [See Exploring Functions With The Use of Manipulatives.] In yet other situations, manipulatives slow the process and should not be used. Remember, manipulatives are made to be outgrown. Below, see how the ( ) are used to encircle the OF information, see which situations are appropriate for manipulative use.  For example: 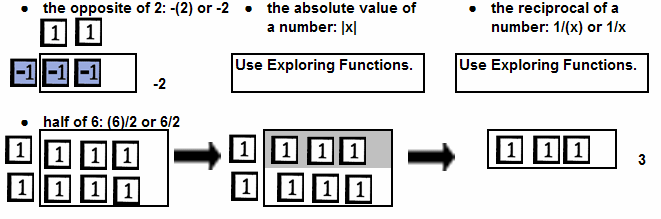
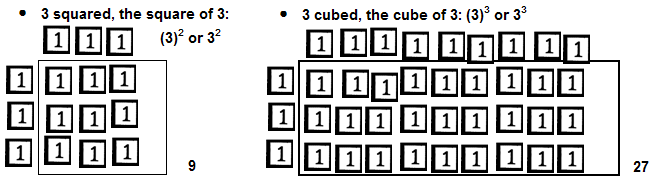
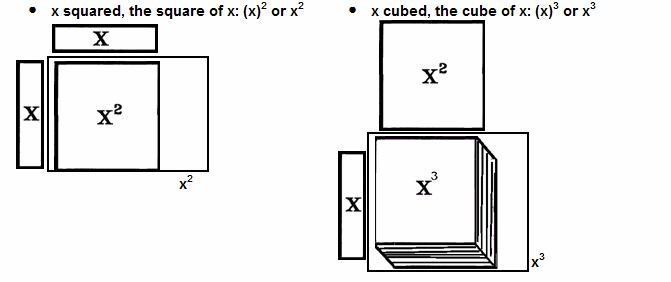

OF is used to sequence the order in which function and operations are to be performed. The objects of a sequences of prepositional phrases correspond to nested sets of parentheses in an algebraic expression -- bottom-most first <=> inner-most first.  Examine the chain of operations and functions that leads to the above different results. 
The only time the opposite of the square of a number equals the square of the opposite number is when the number is 0. To prove this, solve the equation "The opposite of the square of a number equals the square of the opposite of the number." To solve this quadratic equation: collect all terms on 1 side leaving 0 on the other; factor; set each factor to 0; solve each equation; verify solutions. See other examples at "Solve Quadratic Equations." 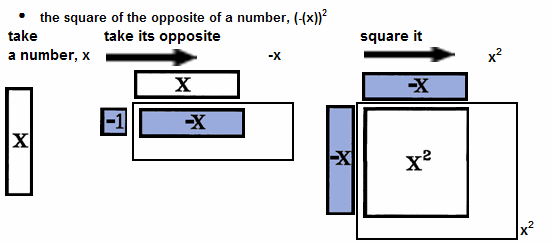 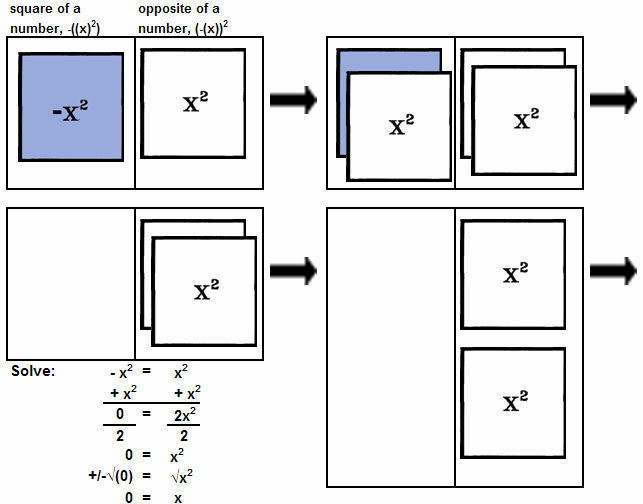
"The square of a number" and "double a number" are another pair of expressions which are often confused. 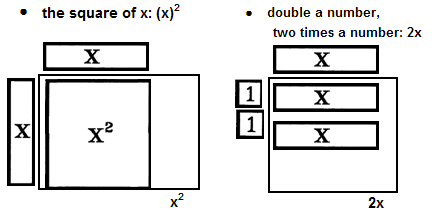
To know when they represent the same thing, solve the equation, "The square of a number equals double a number." 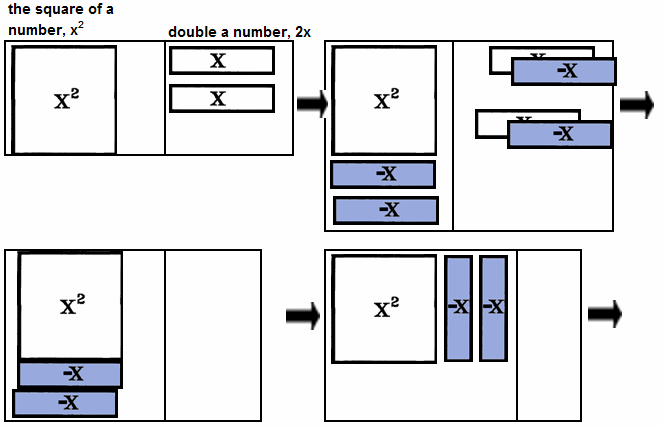 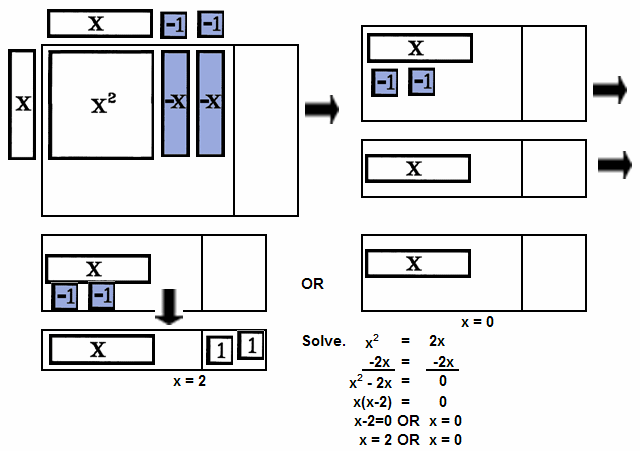
|
 
 www.termtiles.com, Unit 9 © 2008, A. Azzolino |