Term Tiles are terrific tools for teaching algebra and prealgebra. The most basic tiles, labeled on the front, with 1, x, x², and x³, x⁴ have backs printed with -1, -x, -x², -x³, and - x⁴ . They are made to be manipulated, handled.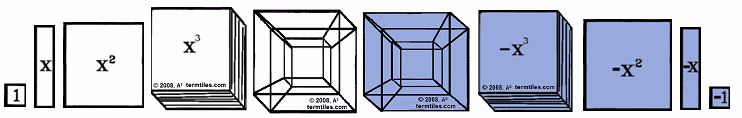

• Complete integer computation.
• Write algebraic expressions in one variable.
• Simplify algebraic expressions in one variable.
• Evaluate algebraic expressions in one variable.
• Complete algebraic computation.
• Factor and distribute.
• Solve linear equations and inequalities and quadratics.
• Solve word problems.
• Complete rational (fraction) computation.

• Simplify algebraic expressions in one and two and three variables.
• Evaluate algebraic expressions in one or two or three variables.
• Complete algebraic computation in one or two or three variables.
• Factor and distribute.
• Solve systems in two variables.
• Examine binomial sums and differences and their squares and cubes.
• Examine the Binomial Expansion and Pascal's Triangle.

      In order to do these things, tiles are chosen for their meaning (representation) then moved or manipulated to achieve the desired goal (operation).

      Term Tiles are manipulatives. In order to maximize use, comments about manipulatives and math are appropriate.

      Term Tiles are tokens or models or toys which are handled and moved and manipulated. They are used to express mathematical ideas and complete computation. Today's manipulatives and tokens come from a long history of human use.

      Tokens were used long before man used cumiform or script on clay tablets or papyrus. They predate written notations and served for thousands of years as a way to tally bushels of wheat and heads of cattle. Writing eventually replaced the tokens (as written algebra will replace manipulative algebra) but not before thousands of years of productive use.

      For the next five thousands years, toys and tokens were used by children to dream and create and play and by generals and admirals to model soldiers and war ships on maps when planning battles. During much of the 19th century, tokens and manipulatives were limited to fraction disks and building blocks (pre solid geometry tools) found in the lower elementary grades.

      More recently spray-painted lima beans, and Hundreds Boards, and Hands-on-Equations®, and Algebra Tiles©, and Graphing-With-Manipulatives© -- modern manipulatives -- found a place in mathematics classrooms.

      Now Term Ttiles are used for counting, and integer computation, and algebraic computation, and equation solving.

      Manipulatives are designed to be outgrown.

      They are thought made concreted. They are the least sophisticated, yet profoundly powerful, computation and representation tool.

      Manipulatives provide "room to think" by expanding both storage and work space to the size of the table top. Think of a generals planning battles involving thousands of soldiers and vast amounts of equipment over hundreds of acres or square miles and the assistance tokens and toy soldiers and maps provide in that situation. Manipulatives work the same way.

      With manipulatives, one can juggle thoughts and tokens before or instead of putting pen to paper.

      Manipulatives may introduce and reinforce ideas from simple to complex, provide flexibility, and encourage experimentation.

      Yet, once mastery and insight are achieved, manipulatives should be discarded. This means Term Tiles are not meant to have a permanent place in a math classroom, but are extremely appropriate and valuable for use at certain times.

      Once the mastery in representation and computation is achieved or it becomes more easy to complete the problem by written or mental work, Term Tiles should be sidelined.

      Before considering logistic suggestions about manipulative and Term Tile use, consider the bigger picture. Think of manipulative use in language acquisition -- achieving mathematical literacy. Think also of communication and representation from the most concrete (manipulative), to the more sophisticated pictorial, to even more sophisticated and abstract formats or the written and spoken word and symbol.

      When acquiring a language, one grows through many stages -- and not necessarily sequential stages. Call them, from the least sophisticated: repression, representation, operation, creation, interpretation. Let's say algebra acquisition is similar to language acquisition and one also progresses through these stages. So, here we discuss the acquisition of the language of manipulatives for algebra.

      In repression, one represses, fights, language use. One says, "It's substandard communication. I can say it better my way."

      Suggestion: Don't force anyone to speak a "less sophisticated language." Use it yourself. Speak it to them. Do not force them to reply using that language.

      In representation, one represents, names, identifies stuff. One asks, "How do you say/represent this or that?" One speaks of things (nouns) like equation, expression, exponent, "one more than a number," "double a number," "the square of a number."

      Suggestion: Have the students do the talking and the teacher do the listening. Have students show things they can represent. Have the student ask a class to represent an expression like "a number decreased by two" or an equation like "A number decreased by two is the same as six." Then have the class represent and solve as needed. Have students use other languages simultaneously -- have them manipulate and draw a picture of their work and write its algebraic code and speak aloud the algebraic code and then translate the code into words.

      In operation, one operates, does things. One asks, "What can I do with this?" or "How do you do this?" One speaks of doing (verbs) like solve an equation or simplify an expression.

      Suggestion: Provide substantial and varied work in both representation and operation in manipulative and more abstract formats and let creation begin!

      In creation, one creates, makes and does original stuff. One says, "Watch/Listen to what I can do," and "See how well I speak & understand." One embraces the language and uses it for one's own purpose rather than because someone else has created a task.

      Suggestion: Have students draw pictures; write test questions and test answers; and teach the class before a test how a procedure is done manipulatively and in algebraic notation. Have one student teach new material to another.

      In interpretation, one interprets or translates one language to another. One says, "Which language suits my purpose best?" or "Should I say the same thing in both/all languages?" and "It doesn't mean exactly that: it's means this."

      Suggestion: Introduce in the concrete (manipulative). Reinforce and review in the abstract (pictures, written and spoken words and symbols). Remember, a good story is worth retelling. Tell it in as many languages or formats as possible.

• Introduce with the concrete (manipulative). Summarize with the abstract (pictures, written and spoken words and symbols).
   
• Introduce a topic with manipulatives. Set the stage for written work by using manipulatives to explore, justify, or explain the topic.
   
• Begin with free play & unstructured time before setting the stage for work.
   
• Create a lesson with beginning, middle, and end. State the goal or objective or the still to be mastered.
   
• Conduct the activity or complete the problem set. Then conclude with a debriefing or summary. Permit the learner to grow beyond concrete thought.
   
• Repeating an exercise set or activity with notes books at hand but manipulatives to the side permits the focus to remain on the writing or abstraction but provides support with the manipulatives if needed.
   
• Keep manipulatives close at hand, perhaps in each student desk, rather than locked in a closet. The easier they are to reach, the easier they are to use.
   
• After use, check one last time on the floor for un-stored pieces.
   
• Debrief yourself at the end of a lesson. Write notes on logistics and educational strategies. List strengths and weaknesses of the lesson. Reconsider the work that might have come before the lesson and plan what work should come next and what tasks still need more work.
   
• Before long term storage write notes on logistics and educational strategies that will make next year's work with the manipulatives even better.